Abstract
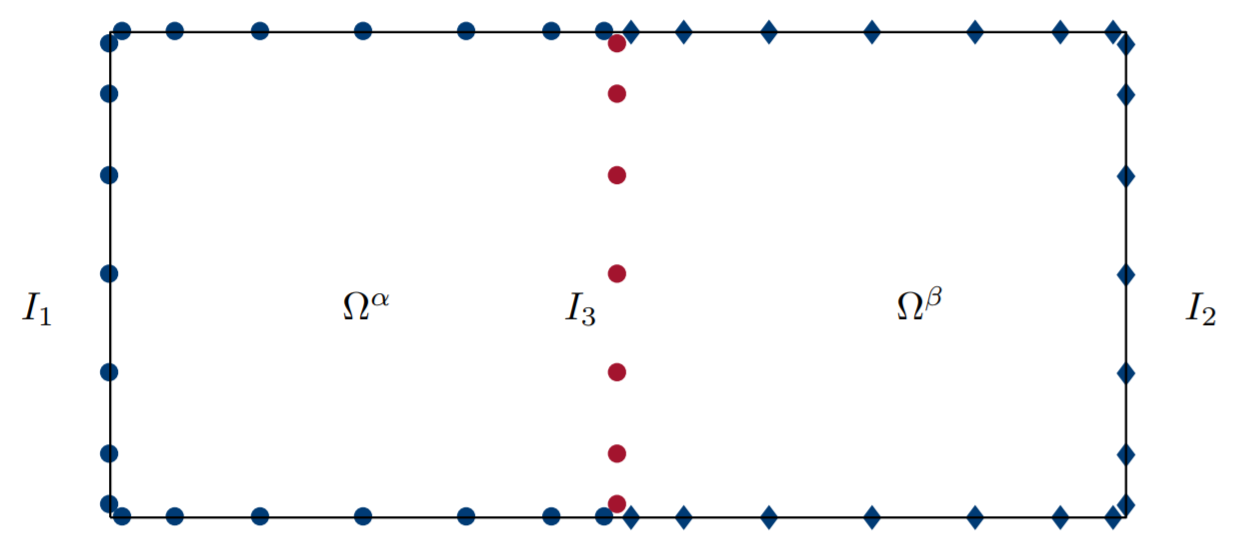
The recently developed Hierarchical Poincaré-Steklov (HPS) method is a high-order discretization technique that comes with a direct solver. Results from previous papers demonstrate the method’s ability to solve Helmholtz problems to high accuracy without the so-called pollution effect. While the asymptotic scaling of the direct solver’s computational cost is the same as the nested dissection method, serial implementations of the solution technique are not practical for large scale numerical simulations. This manuscript presents the first parallel implementation of the HPS method. Specifically, we introduce an approach for a shared memory implementation of the solution technique utilizing parallel linear algebra. This approach is the foundation for future large scale simulations on supercomputers and clusters with large memory nodes. Performance results on a desktop computer (resembling a large memory node) are presented.
